The Common Core State Standards Initiative (CCSSI 2010) states, “The Standards for Mathematical Practice describe varieties of expertise that mathematics educators at all levels should seek to develop in their students” (p. 6). College instructors rate the Mathematical Practices as being of higher value for students to master in order to succeed in their courses than any of the CCSS content
standards. This is true for mathematics, language, science, and social science instructors (Conley, et al. 2011).
Specifically, the Mathematical Practices build on the recent work of NCTM (2000, 2006, 2009) and the National Research Council (Kilpatrick, Swafford, and Findell 2001) and emphasize the learning of mathematics as much more than procedural knowledge. The groundwork NCTM and the National Research Council laid is reinforced and further
refined in the CCSS Mathematical Practices.
As you seek to make sense of the Mathematical Practices and the mathematical content
standards contained in the CCSS for mathematics, take advantage of this opportunity to develop with your colleagues a collective understanding of effective and meaningful teaching of mathematics at your high school. This article provides one example designed to help you address Mathematical Practice 4: Model with Mathematics.
According to Kanold, et al. (2012), it is a good idea for you and your colleagues to ask and answer three critical questions for each of the Mathematical Practices:
- What is the intent of this CCSS Mathematical Practice, and why is it important?
- What teacher actions facilitate this CCSS Mathematical Practice?
- What evidence exists that students are engaged in this CCSS Mathematical Practice?
What is the intent of Mathematical Practice 4?
Mathematically proficient high school students are able to use their knowledge of mathematics to model various phenomena or mathematical ideas. Modeling involves the ability to see mathematics as a way to make sense of both the world around us as well as the world of mathematics.
In any high school course, students engaged in CCSS Mathematical Practice 4: Model with mathematics solve real-world problems by applying known mathematics (CCSSI p. 7). In this practice, students might use tools such as diagrams, tables, graphs, graphing calculators, and area or volume models to make sense of the mathematics. More generally, students use symbols and tools to represent real-world situations and move fluidly between different representations based on the questions they are trying to answer.
Although the ways students model and represent situations will evolve as students learn more mathematics, this should be an expectation for the student experience in every chapter of every high school course. A goal is for students to model mathematics often in order to become more mathematically proficient at this practice.
Teachers must give high school students the opportunity to explore real-world problems or situations and then encourage them to represent those problems mathematically. Once students can represent a problem with mathematics, they can then solve the problem and interpret their results within the context of the problem. All this depends on the students being provided the opportunity to solve problems that arise from everyday life. These sorts of experiences will prepare students for expectations related to reasoning mathematically in high school (NCTM 2009).
What is your role as the mathematics teacher?
Your role is to provide opportunities for students to explore and share solutions to real-world situations
that present themselves in and out of daily school life. Thus, your classroom environment must be conducive to peer-to-peer discourse.
Students engaged in this Mathematical Practice will not only check for reasonableness of the computation(s), but will extend sense making to determine if the solution is appropriate given the original problem context. You will need to support this practice by asking students if their answers are reasonable, facilitating the classroom discourse that allows students to learn from each other, and ensuring that students make sense of their answers according to the context of the problem.
By providing opportunities for students to develop real-world contexts to correspond to mathematics equations and then to check to make sure the correspondence is accurate, you facilitate students’ sense making relative to modeling mathematics.
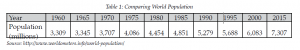
One such student problem task involves using functions to model world population data, including linear, quadratic, and exponential functions. Students can use a graphing calculator or a software tool to examine a set of data and determine the best-fitting model from a list of familiar functions. Consider the
world population (in millions) from 1960 through 2015, as shown in Table 1. Your students could create a scatterplot of the data and then use the regression capabilities of the graphing calculator or computer software to determine the best-fitting model for the data. You can go to p. 14 Figure 1.4 of Beyond the Common Core: High School (2015) for a reproducible version of this table and the complete problem task for use in class—including extensions of the problems.
As your teacher team develops or identifies mathematical tasks in each chapter that require students to model contextual problems and construct viable arguments to support their reasoning, you should work together to anticipate what students will do, to determine what constitutes a viable argument, and to teach students how to use a tool (such as a graphing and statistics tool) strategically. The mathematical practice of Model with Mathematics begins with you as you and your colleagues commit to the routine use of tasks like these that provide students an opportunity to use models from real-life situations.
References
Common Core State Standards Initiative (CCSSI). 2010. Common Core State Standards for Mathematics. Washington, D.C.: National Governors Association Center for Best Practices and the Council of Chief State School Officers. www.corestandards.org/Math.
Conley, D., K. Drummond, A. Gonzalez, J. Rooseboom, and O. Stout. 2011. Reaching the Goal: The Applicability and Importance of the Common Core State Standards to College and Career Readiness. Eugene, OR: Educational Policy Improvement Center. www.epiconline.org.
Kanold, T., D. Briars, and F. Fennell. 2012. What Principals Need to Know about Teaching and Learning Mathematics. Bloomington, IN: Solution Tree Press.
Kanold, T., et al. 2012. Common Core Mathematics in a PLC (K–12): High School. Bloomington, IN: Solution Tree Press.
Kilpatrick, J., J. Swafford, and B. Findell, eds. 2001. Adding It Up: Helping Children Learn Mathematics. Washington, DC: National Academy of Sciences.
National Council of Teachers of Mathematics (NCTM). 2006. Curriculum Focal Points for Prekindergarten through Grade 8 Mathematics: A Quest for Coherence. Reston, VA: NCTM.
2009. Focus in High School Mathematics: Reasoning and Sense Making. Reston, VA: NCTM.
2000. Principles and Standards for School Mathematics. Reston, VA: NCTM.
[author_bio id=”390″]